Some of the newer belt designs require greater initial tension to achieve their performance claims. But these higher tensions may produce unwanted effects, such as tension excursion. Here’s a closer look at what you can do to minimize these effects.
If you select the proper pitch, tooth profile, and component materials based on an application’s torque capacity, drive speed, and positioning accuracy, you will probably have a reliable drive system that will yield millions of trouble free operational cycles. Selection, however, is only one step. Proper installation is necessary to avoid premature failure of the drive system or its components. Part of proper installation involves runout control. How you attach sprockets to the shaft can play a key role in reducing tension excursion and runout.
It’s in the teeth
Synchronous belt drives with teeth arranged perpendicular to the belt’s pitch line were first developed in the 1940’s as a more efficient alternative to v-belt drives, which had a tendency to slip in operation due to several factors, including the fact that they stretch after initial installation and tensioning.

First generation synchronous belts featured trapezoidal tooth forms and delivered positive drive and greater efficiency than v-belt drives. Under load, the teeth mesh with the mating pulley teeth at the pulley’s tooth root radius and the upper flank of the pulley tooth on the tight side of the belt.
Second generation synchronous belt drives are dubbed ‘high torque drive’ and use a curvilinear profile for the belt and sprocket teeth.
The first-generation synchronous-belt tooth profile had a trapezoidal tooth form, which is still a popular choice to this day, and provides positive drive and greater efficiency than v-belt drives. When under load, the belt’s teeth mesh with the mating pulley teeth at two points, the pulley’s tooth root radius and the upper flank of the pulley tooth on the tight side of the belt.
Clearances were designed into the mesh to ease entry and exit of belt tooth to pulley groove thus reducing noise, vibration, and wear. Some of the compromises of this design, though, include limited load-carrying capability due to the low flank angle; a tendency to ratchet or jump teeth when the tension is too low or the load is too high; and accelerated belt wear at the stress risersgenerated at the contact points between belt and pulley teeth.
The second generation of synchronous belt drives is currently marketed by Gates Corporation, and dubbed HTD®, an acronym for ‘high torque drive.’ This belt design uses a curvilinear profile for the belt and sprocket teeth. Variations on this theme are now offered by many of the belt drive manufacturers.
The designs have the advantage of a deeper tooth form, which allows substantially higher torque transmission and a larger area of contact between the tooth and belt under load. It also mitigates the high stress areas inherent in the trapezoidal designs. The compromise for the greater torque is that larger clearances are required for smooth tooth entry and exit into the mesh, which leads to greater backlash between the mating surfaces. The backlash may eliminate this tooth profile from consideration in applications that need accurate positioning.
In the early 1980’s a modified version of the curvilinear design emerged, which modeled its tooth shape on the involute and trochoid curves found in gear teeth. As a result, the clearances required for smooth entry and exit into the mesh were greatly reduced and a larger contact area distributes stresses more evenly, allowing greater loading capacity.
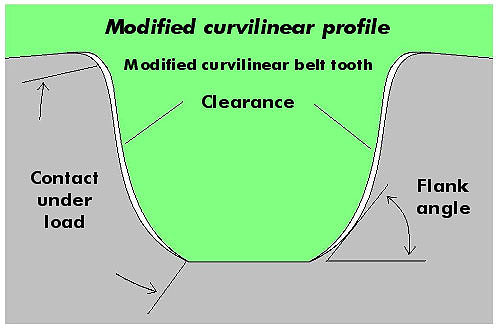
A modified version of the curvilinear design modeled its tooth shape on the involute and trochoid curves found in gear teeth. This design reduced the clearances required for smooth entry and exit into the mesh. The larger contact area distributes stresses more evenly, allowing greater loading capacity.
The current state of the art improves on the positioning accuracy available with trapezoidal designs–up to 40% more torque capacity than the original curvilinear designs. Plus, trapezoidal and original curvilinear designs max out at 10,000 rpm. The modified curvilinear designs max out a 14,000 rpm — a 40% increase in speed. The newer curvilinear designs also feature lower noise, greater ratcheting resistance, and increased power density. Current debate within the development community centers around whether the improvements deserve a designation of third generation, which some engineers argue should be reserved for an order of magnitude advance in technology, rather than a incremental upgrade.

Several methods exist that support the motor shaft to prevent internal bearing damage when press fitting a component. However, these methods all have drawbacks. A newer method uses a single screw to attach the component to the shaft. It can be phased repeatedly without damage or loss of torque capacity and well suits applications requiring precise runout control.
So, what’s the catch?
The newer designs require a higher initial tension to achieve some of the performance claims due to the higher modulus tensile cord materials and more rigid elastomeric compounds used in their construction. While some original designs used polyester cord, more recent versions use fiberglass or aramid (Kevlar®) fiber tensile cords, which exhibit significantly less stretch or give under load.
The higher initial tension is well tolerated on a static basis but may produce undesirable, even intolerable forces when the system is put into motion. The dynamic forces at issue here are defined as tension excursion (TE) and occur when component eccentricity during assembly is not carefully controlled.
Engineering standards define requirements for runout control for components but fail to address the dynamic aspect of systems in motion. To quantify tension excursion you can use the equation:
TE = 2TI (Δ CD)/PL
Where:
TE = tension excursion, lb.
TI = initial belt tension, lb. (static)
Δ CD = chage in center distance, in.
PL = belt pitch length, in.
Note that the magnitude of TE is inversely proportional to belt pitch length, and that shorter drive-center distances will exhibit higher tension excursions when expressed as a percentage of initial tension. The formulas also assume worst case with the center distance change or total runout multiplied by two.
Best case, the TE phenomenon will be random when using equal diameter pulleys, and luck may run with you when a drive is assembled and the eccentricities are in synch and cancel each other out. But Murphy’s law will often prevail, and a conservative approach will assume that the eccentricities will run opposed and max out the tension. The TE will surely appear and be a cyclical phenomenon when using integral or hunting ratios in a drive train.
The effects of excessive TE
Depending on the magnitude of the TE, and how conservative or aggressive the initial drive design, problems can range from belt damage, to premature bearing failure, to reverse bending fatigue failure of the shaft, to motor rotor clearance issues, as well as other problems that may or may not be readily apparent or attributable to TE. The bottom line is that if you lessen tension excursion, all of the components in the drive will last longer, have less vibration, be more accurate, and will be able to run faster if desired.

Clearances required when a pulley is attached to a shaft tend to be shoved to one side of an assembly when setscrews are tightened. Thus, the entire clearance is added to radial runout.
Removing runout
Runout exists in all the drive components, including shafts, bearings, motors and sprockets or pulleys. The most practical component to address is the pulley or sprocket, since it is often the largest contributor to total runout as assembled.
Improvements in machine accuracy and manufacturing methods allow component suppliers to provide product that is held to a closer static runout accuracy than the Rubber Manufacturer’s Association (RMA) and Mechanical Power Transmission Association (MPTA) engineering standards dictate, and this is a good start.
However, machine and drive designers must also consider the method used to attach the sprockets to the shaft, as this is where good intentions can often go off course. The most common method of shaft to hub attachment is two set screws at 90º. To quote Dr. Woodie Flowers, Professor Emeritus and Director of the New Products Program at MIT’s Mechanical Engineering Department, “Setscrews suck.”
Consider the nature of a pulley attached to a shaft using two setscrews at 90º. The design is driven by the requirement to easily fit the pulley to the shaft, so typically a clearance of 0.001 in. to 0.003 in. is specified between the shaft at maximum material condition and the bore at minimum. Whatever clearance exists between the shaft and bore is going to be shoved to one side of the assembly when the setscrews are tightened, so that the entire clearance will be added to radial runout. Again, Murphy may not show up and the two setscrews might exactly oppose the static runout and cancel out the assembly eccentricity. In either case, the desired result is left to chance. A trial fit and adjustment might be in order, but then again, there’s those pesky setscrew burrs on the shaft to deal with.
Putting it all together
A press fit between components is economical, but often not practical. You must use a method to support the motor shaft to prevent internal bearing damage when press fitting a component.
Adjustment after assembly is impractical. A pinned assembly with a specification callout of close tolerance is often impractical due to the additional cost and uncertainty of assembled fit. Again, adjustment is not practical.
The usual answer is to use rotary hub-to-shaft connections, the best of which use opposing tapers to evenly apply pressure to both the hub and shaft and accomplish a mechanical shrink fit as assembled. But not all of these tapered connection devices are created equal. Many require extra tools, multiple screws, and additional space. The devices are often separate from the component being attached and therefore require additional assembly.
A newer connection system uses a single screw, which never touches the shaft, to force a tapered bushing into place, creating a mechanically shrink fit between the component and the shaft. Such a design can be phased repeatedly without damage or loss of torque capacity and better suits applications requiring precise run out control.
Custom Machine & Tool Co., Inc.
www.cmtco.com
::Design World::
Filed Under: Belts + chains, MECHANICAL POWER TRANSMISSION





Tell Us What You Think!